珍珠湾ART

标题: 证明五园共点 [打印本页]

作者: 野菜花 时间: 2006-11-28 23:50
标题: 证明五园共点

平面上有5个园，其中任4个园都至少有一个共同点，证明这5个园至少有一个共同点.

作者: HF: 时间: 2006-11-29 05:50
标题: 回复：证明五园共点

Every two circles 'share' a staight line -- the one pass through the two intersection points or the tangent line if the two circle only share one point.

Eanch of circle 1,2,3,4 'shares' a line with circle 5, and by condition, every 3 of the four lines share one point -- and from this, we can show that these four lines must share the same point, and that must be the point share by all circles.

作者: 野菜花 时间: 2006-11-29 18:43
标题: Good! [:-Q][@};-]

Good!

作者: xlxk 时间: 2006-11-29 19:39
标题: 回复：回复：证明五园共点

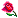
如果这儿所说的圆只是圆周的话，这个证明正确。

如果是包含圆周和圆周包围的圆面的话，这个命题仍然成立，证明要难很多！

作者: 野菜花 时间: 2006-11-29 22:17
标题: 回复：回复：回复：证明五园共点

我的题目是指园周，你说对园面也对但证明难得多，证明有趣吗？欢迎另开一题和大家分享！

作者: 野菜花 时间: 2006-11-30 20:45
标题: 另一个证明

设五个园为abcde，

设A点为四园bcde 的一个公共点， B点为四园acde 的一个公共点, 等等.www.ddhw.com

如果A，B，两点重合，这重合点既是bcde 的公共点又是 acde 的公共点所以是五园的公共点。

所以可设A，B，C 三点不同，两园 d,e 都过这三点，所以园 d,e 重合，这样五园abcde 的公共点就是四园abcd 的公共点。

欢迎光临珍珠湾ART (http://art.zhenzhubay.com/)